John Washington Allen (1803 - 1856) was
the son of cousins and may have married his
own (double) second cousin (consanguinal degree 4).
How closely are two people related? Some interesting such questions
arose while plotting my pedigree.
Here's a simple introductory table that's well known:
| Degree 1 | Degree 2 | Degree 3 | Degree 4 | Degree 5 | |
|---|---|---|---|---|---|
| parent's generation | father | uncle | half-uncle | 1st cousin 1x removed | 1st half-cousin 1x removed |
| same generation | brother | half-brother, double-cousin | 1st cousin | 1st half-cousin | 2nd cousin |
| child's generation | son | nephew | half-nephew | 1st cousin 1x removed | 1st half-cousin 1x removed |
| grandchild's generation | double grandson | grandson | grand-nephew | grand half-nephew | 1st cousin 2x removed |
| gene-sharing probability | 1 / 2 | 1 / 4 | 1 / 8 | 1 / 16 | 1 / 32 |
The table shows, for example, that one is equally close genetically to one's half-uncle and one's first cousin, though they are of different generations. If you have a rare gene, your half-uncle or first cousin has a 1/8 chance of sharing that gene. (Here and throughout this article we assume the rare gene is due to inheritance, not mutation.) For brevity, the table just shows male relations, but an aunt or niece is just as close as an uncle or nephew. (A child does receive somewhat more genetic material from its mother than from its father, but this is ignored.)
Closeness is symmetric, as seen by comparing "parent's generation" and "child's generation" in the table.
With two exceptions, only very simple relationships are shown in this table. (Half-siblings, half-cousins, etc., which arise when a common ancestor had children by two different spouses, are actually simpler than full-cousins.) The exceptions are "double-grandchild" and "double-cousin" which I've included in the table just to remind the reader that more complicated cases are possible. The former involves incest --- the illicit mating of close relations. This may be an unpleasant subject, but marriage of royal siblings was common in some ancient cultures; for example King Darius II of Persia married his half-sister, after murdering his half-brother King Sogdianus. (Don't feel too sorry for Sogdianus: he acquired the throne by murdering his half-brother King Xerxes II.) Many more recent cultures do not treat marriage of first cousins as illicit. When half-siblings (or full-siblings) mate and have a child, the one (or two) person who is both the child's paternal grandparent and maternal grandparent has the same genetic closeness as a parent.
The other exception, "double-cousin," does not involve the mating of blood relations. (A man who marries his brother's sister-in-law is not violating a taboo or allowing genetic degradation.) When two siblings marry two siblings, the offspring are double-cousins. There are several pairs of double-cousins who are both ancestors of mine; among the most famous double cousins are two 13th century Kings. A variety of other complications are possible, but the most common complicated relationships do involve incest.
The reason I created this web page is to show how to calculate consanguinal distance.
Suppose I inherited a very rare gene from one of my parents. What is the chance my sister also has the rare gene? One-half. What is the chance my half-sister has it? One-quarter. (This assumes I don't know which parent I inherited the gene from, but that's the right assumption since we're actually concerned with many genes.) If we take the logarithms of one-half and one-quarter, always using the base one-half, we get 1 and 2. These are the consanguinity degrees of sibling and half-sibling shown above, and this is the mathematical model we adopt.
Let's compare my nephew and me. My brother has a one-half chance of sharing my rare gene and there's a one-half chance he passed it to his son (my nephew). That works out to a one-quarter chance; log(1/2)1/4 = 2. Let's try the other way. If my nephew has a rare gene, there's a one-half chance he got it from my sibling, and then a one-half chance I share it with my sibling. Again, a net chance of one-quarter.
There is a much simpler way to calculate this answer. Find the chains connecting two people using common ancestors, and see how long they are. Between my nephew and me there are two such chains, using my Mother and Father as the common ancestors: Me --> My Mom --> My sibling --> My nephew and Me --> My Dad --> My sibling --> My nephew. Each chain is of length 3.
With two or more chains, we will have to "sum" them somehow to compute the consanguinal distance. With a single chain, we can read the distance directly: Between me and my half-nephew there is a single chain of length 3 so our consanguinal distance is three. Between me and my half-cousin there is a single chain of length 4 so our consanguinal distance is four.
Between me and my full-nephew there are two chains of length 3 and, using the peculiar arithmetic 3 + 3 = 2, our consanguinal distance is two. That peculiar arithmetic makes more sense like this: 2-3 + 2-3 = 2-2.
In the preceding I assumed there's a one-half chance that one's sibling or child shares one's rare gene. Let's see if that's true.
In the "haploid" genetics model, a person inherits an allele with equal probability from either parent. Then my child has a 50% chance of inheriting my gene. I don't know which parent I inherited it from but we can assume only one parent has the gene, since we said it was rare. Whichever parent it was, my sibling had a 50% chance of inheriting it. The assumption checks. Remember: a person's chance of having the rare gene is the mean average of the such chances of his two parents. This more general rule lets one calculate consanguinity even in complicated cases involving interbreeding.
But that's not the way genetic inheritance works: higher life forms are "diploids," not "haploids." Each parent has two alleles, or two opportunities, for a given gene, and I inherit one allele from each parent. Call the rare gene X and its normal form O. One of my parents is XO and the other OO (because the gene is "rare"); I inherit from both parents, but only one allele each. Again the chance of acquiring "X" is 50%.
We get the same answer, even though the reason is completely different. If you compute the consanguinal distance of cousins, 2nd cousins 1x removed, great-great grand-nephew, or even double-cousins you will get the same answer using either of the mathematical models mentioned above, even though one is "right" and one is "wrong."
With more complicated relationships involving incest, the two procedures give different answers. Unfortunately, the simple method based on counting parent-child links gives the answer for the wrong mathematical model.
As far as I know, all genealogists who study consanguinity follow the haploid model and so will we. It is much simpler to compute, gives the correct answer when there's no interbreeding, and gives a useful approximation even when there is interbreeding. In addition to being hard to compute, the diploid distance defined in terms of gene-sharing probability has a fundamental flaw: the consanguinity distance from A to B is not generally equal to the distance from B to A. (If the reader knows a better way to compute diploid consanguinity distance, please e-mail me.)
One special case requires special mention. Identical twins are treated as though they are the same individual: their gene-sharing probability is 1 and consanguinal distance 0. The children of fraternal twins are cousins (consanguinal distance 3) but the children of identical twins have consanguinal distance 2. When identical twins marry siblings their children have consanguinal distance 1.415; if the sibling spouses were also identical twins the "double cousins" have distance 1.
The most incestuous marriage in my family tree is that of the parents of Mahalalel. This provides a good exercise to compute consanguinity. Nevermind that the people are mythical: this might be the real family tree of some Adam Smith and Eve Jones that got stranded on a deserted island.
Some of the medieval noble families interbred and one wonders if the unions were incestuous. Of course there's no accepted definition of incest. (The Church of England publishes a list of proscribed marriages: according to it a man may marry his first cousin, but not his son's widow. Genetic degradation can't be the worry here, since the latter is not a blood relation.) Today, many people agree that first cousins (consanguinity 3) shouldn't marry and have children, and that for second cousins (consanguinity 5) or beyond there should be no incest qualms. What about the marriage of first cousins 1x-removed (consanguinity 4)? We'll arbitrarily call that the threshold of incest. (Does anyone know if it's actually outlawed anywhere?) We have no ill intent with this definition, although the maternal grandparents of England's present Prince Consort Philip, Duke of Edinburgh, had that relation. (Speaking of Philip, his ancestry is so steeped in royal blood that he was chosen, among all living descendants of the Russian Czars, as the best fit for a DNA test with the Anastasia imposter.)
There are so many examples of ancient nobles marrying their nieces or half-sisters, I shouldn't bother to mention any that "just" marry first cousins, or even the child of cousins who married a cousin, but there's one interesting example of the child of cousins marrying a cousin where, due to extra links, the consanguinity distances are 2.61 and 2.79 instead of the usual 3.00 for cousins.
Finally I mention one more example where the child of cousins married his own double second cousin. Unlike the other examples, taken from medieval Europe and shown on many family trees, this relationship involving my Allen progenitors may not be documented anywhere except on this family tree.
John Washington Allen (1803 - 1856) was
the son of cousins and may have married his
own (double) second cousin (consanguinal degree 4).
I say "may have" because even though one Internet source shows that John W. Allen had the double second cousin, Martha Givens, born in Kentucky about 1803, and our own family records show that John Washington Allen married a Martha Givens born about the same year also in Kentucky, I don't yet have any positive evidence that the two Martha Givens are the same.
UPDATE 2016: A distant cousin found via Ancestry.com's DNA matching
now essentially confirms the Givens connection shown in my tree!
Assuming Adam and Eve were genetically unrelated individuals, their children were of consanguinity degree 1 to each other and to their parents -- nothing out of the ordinary here -- but what about Adam and Eve's grandchildren? The degrees will no longer be whole integers so it's better to work with the (haploid) gene probabilities directly. p(Seth, Seth) = 1 and p(Seth, Azura) = 1/2, so p(Enosh, Seth) = 3/4. The same arithmetic works for the other three 2nd/3rd generation combinations. This answer can be obtained by counting links in the common ancestor chains as well: Enosh --> Seth ; Enosh --> Azura --> Adam --> Seth ; Enosh --> Azura --> Eve --> Seth. There is one chain of length 1 and two chains of length 3, and 2-1 + 2-3 + 2-3 = 3/4 ~= 2-.415. The consanguinity degree between Enosh and his father is 0.415; you can check easily that this is also the degree between Enosh and his sister. Fractional consanguinity may seem confusing, but from a practical standpoint the consanguinity less than 1 means that "something has gone wrong and, anyway, you'd better stop inter-marrying now!"
The chains we listed may seem wrong (you're supposed to go back just to the least distant common ancestor) but the chains that bypassed Seth used his wife/sister independently so they're correct and give the correct (haploid) answer. The requisite chains for the Enosh-Noam connection are: Enosh --> Seth --> Noam ; Enosh --> Azura --> Noam ; Enosh --> Seth --> Adam --> Azura --> Noam ; Enosh --> Seth --> Eve --> Azura --> Noam ; Enosh --> Azura --> Adam --> Seth --> Noam ; Enosh --> Azura --> Eve --> Seth --> Noam. Now the multiple overlapping chains seem even more wrong, but we're still really OK --- no person appears twice in any chain.
In the same fashion we can derive p(Cainan, Enosh) = 7/8, and p(Cainan, Mualeleth) = 7/8. To demonstrate the latter, with our "chain arithmetic" we have to show 14 chains: 2+2+4+4+4+4+6+6+6+6+6+6+6+6. It is possible to do that with the restriction that no individual appears twice in any chain. To see this, note that there are four ways to get from Cainan to Adam (Adam is Cainan's "quadruple great-grandfather") and in each case we can get back to Cainan's wife/sister using different individuals. That provides 4 chains of length 6, the other 4 use Eve; the chains of length 4 use Seth and Azura as double-grandparents and the chains of length 2 are the ordinary chains all siblings have.
This entire discussion is for the "haploid" model. The real ("diploid") gene-sharing probabilities are somewhat different.
There are several pairs of double-cousins who are my ancestors. Count Alan III of Brittany and his brother Count Eudes ``Penthievre'' are double-cousins with six of my ancestors, namely the children of their maternal uncle, Richard II ``the Good,'' Duke of Normandy, since he married their paternal aunt, Judith, Duchess of Brittany.
There are seven other pairs of double-cousins in my pedigree. The most ancient of these is Swinthila, King of the Visigoths, a double-cousin of Athanagild II of the Visigoths. The most recent is Henry Beaumont, a double-cousin of Blanche of Lancaster.
Since double-cousins are as closely related as half-siblings, the children of two double-cousins are as related as half-cousins and therefore shouldn't marry. Nevertheless we can find such a marriage in my pedigree. If you check the tree of Jeanne de Valois you will see that her two grandfathers (both of them Kings) are double-cousins. The same statement holds for Jeanne's brother, King Philip VI of France, but he's not in my database since he's not my direct ancestor.
Their parents, by the way, had even closer consanguinity than degree 4. This is because Philip VI's paternal grandmother, Isabelle of Aragon, has a variety of links to the other grandparents, as we see next.
The main incestual problem with Philip VI's heredity is that his
parents were double second-cousins, but in addition his grandmother
Isabelle has blood relationships to each of his other grandparents.
Only the five closest such links are shown in the figure.
Isabelle of Aragon is half-cousin of King Istvan VI, and 2nd cousin of Margarite and Beatrice. Her maternal grandmother is both a 1st cousin and a 2nd cousin of King Louis VIII.
Only individuals required to depict these links are shown. (This is true of all the charts on this page.) When siblings appear in the figure, all parents are shown to distinguish half- and full-siblings.
Finally what is the gene-sharing probability between Philip VI's parents? We need only sum their parent probabilities p(Isabelle, Charles II) + p(Isabelle, Maria) + p(Philip III, Charles II) and divide by four. (Links between Isabelle and her husband don't affect her daughter-in-law and p(Philip III, Maria) = 0.) p(P III, C II) = 1/4 (they're double cousins); p(I, M) = 1/32 (they're half-cousins 1x-removed); p(I, C II) = 1/64 + 1/128 + 1/512. (They're 2nd cousins 1x-removed via King James I; 3rd cousins via Yolande of Flanders; 4th cousins via King Philip I.)
Therefore the gene-sharing probability between Duke Charles III and his wife is 157/2048 (or somewhat more actually due to more remote links not shown). Their degree of consanguinity is about 3.70.
There are numerous instances in my tree where first cousins marry,
but one is worth mentioning while we're on the subject of double-cousins.
If you check the tree of
Agnes de Clare you will see her
two grandfathers are brothers (in fact they are both double-cousins
of Count Alan and Count Eudes as mentioned above).
The parents of King Ordono III
were not only first cousins (their mothers were sisters),
but one of their fathers was a first cousin of those sisters,
and the other father was a second cousin of them.
Ordono III married the daughter of yet a third sister.
Thus the gene-sharing probability between Ordono III's parents
is 1/8 + 1/32 + 1/128.
The gene-sharing probability between Ordono III and his wife
is 1/16 + 1/16 + 1/64 + 1/256.
Adelaide of Alsace
(aka Adelheid von Nordgau) was the mother of
Conrad II "the Salic," Holy Roman Emperor.
Adelaide was the product of incest:
Adelaide's mother, Eva of Luxemburg, was the niece of
Adelaide's father, Count Gerhard of Alsace.
There are many instances of medieval noblemen marrying their nieces;
the reason this example is interesting is that Eva was herself the
product of such an incest: her father Siegfried married his half-niece Hedwig.
As shown in the figure, Adelaide also married a relative, though his closest link is only consanguinity degree 6. Obviously this family wasn't over-concerned about incest taboos, but let's pose the academic question: was Adelaide's marriage to Duke Henry II of Franconia incestuous? Count Gozelon is the uncle of Adelaide's father and both the half-uncle (paternally) and great-uncle (maternally) of Adelaide's mother. Because 2-4 + 2-4 + 2-4 + 2-5 + 2-5 = 2-2, this means Gozelon has consanguinity degree 2 with Adelaide, so his great grandson (Henry II) has consanguinity 5 with her: suitable for marriage.
In several examples of medieval nobility, a pair with closest
single chain of degree K have consanguinity of approximately (K-1) when
many chains are considered.
Here is the list of the Duke of Edinburgh's Great-great Grandparents:
Duke Wilhelm of Schleswig-Holstein, Landgravine Luise of Hesse-Cassel, Landgrave Wilhelm of Hesse-Cassel, Princess Charlotte of Denmark, Czar Nicholas I of all the Russias, Princess Charlotte of Prussia, Duke Joseph of Saxe-Altenburg, Duchess Amalie of Wurttemberg, Grand Duke Ludwig II of Hesse and by Rhine, Margravine Wilhelmine of Baden, Count Johann Moritz, Sophie Lafontaine, Prince Karl of Hesse and by Rhine, Princess Elisabeth of Prussia, Prince Albert of Saxe-Coburg and Gotha, Queen Victoria of the U.K. and Empress of India.
Among the 16 ancestors there is an emperor, an empress, nine other nobles ranked Duke or higher and four nobles with rank equivalent to Earl or Marquis. I don't know how Sophie snuck in, but her father probably had "blue blood" since he had two middle names: Doctor Franz Anton Leopold Lafontaine.
The consanguinal links documented in ancient noble families can be quite complicated. The Dukes of Normandy and King Henry I had many children who formed the early English nobility and tended to marry among themselves. One often sees a nobleman marrying a relative of his mother; in a medieval rural setting there may have been little choice of marriage partners with a high social status.
Here is part of the tree of the Duke of Buckingham. This tree overlaps a preceding tree slightly: the "Duke of Anjou" is the father ("Duke Charles III") of King Philip VI of France.
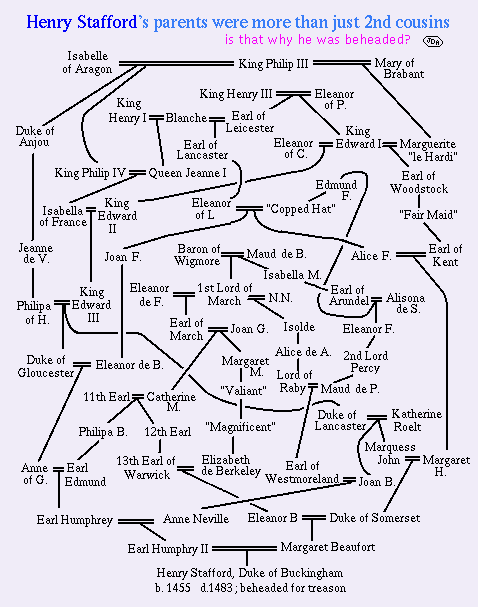
Henry Stafford's parents were 2nd cousins --- they were both great grandchildren of John of Gaunt (Duke of Lancaster) and his wife Katherine Roelt. However they had numerous more distant links as well, many of which are shown in the Figure.
Can you figure out what their degree of consanguinity is? Just assume the links shown in this Figure: nevermind that both Elizabeth de Berkeley's husband and father were descendants of King Edward I by paths not shown, as were Earl Edmund and his mother-in-law Eleanor de B. Nevermind that Earl Edmund is also descended from Isolde, that Earl of March is the 1st cousin of "Fair Maid's" mother, that the Earl of Lancaster's wife was 1st cousin of 11th Earl of Warwick, etc.
If you give up, or want to check your answer, here is the solution to this exercise.
| Here is a key to the persons in the tree:
| ||
|---|---|---|
|
|
|
| ||
| * - Persons listed with an asterisk are not shown on the chart; their position is defined by ahnenreihe number. | ||
Note that all four grandparents, and 6 of eight gt-grandparents were Habsburgs. Here are the computed inbreeding coefficients depending on how far you look back:
(1) Carlos' maternal grandmother was the child of Carlos' paternal grandparents.
4,5 --> 7 --> 3 --> 1 --> 2 --> 4,5 [.125 = 32/256]
(2) Carlos' maternal grandfather was, on his father's side, the nephew of Carlos' paternal grandmother;
24,25 --> 12 --> 6 --> 3 --> 1 --> 2 --> 5 --> 10,11 [1/32 = 8/256]
(3) and, on his mother's side the 1st cousin 1x removed of that same paternal grandmother.
22,23 --> 26 --> 13 --> 6 --> 3 --> 1 --> 2 --> 5 --> 11 --> 22,23 [1/128 = 2/256]
(4) Carlos' paternal grandparents were 2nd cousins; this would not affect Carlos' raw score except for (1).
32,33 --> 16 --> 8 --> 4 --> 7 --> 3 --> 1 --> 2 --> 5 --> 10 --> 20 --> 32,33 [1/512]
32,33 --> 20 --> 10 --> 5 --> 7 --> 3 --> 1 --> 2 --> 4 --> 8 --> 16 --> 32,33 [1/512]
(5) Carlos' maternal grandfather was 2nd cousin of his paternal grandfather.
20,21 --> 10 --> 12 --> 6 --> 3 --> 1 --> 2 --> 4 --> 9 --> 18 --> 20,21 [1/256]
(6) Carlos' maternal grandmother was 2nd cousin of his paternal grandfather.
20,21 --> 10 --> 5 --> 7 --> 3 --> 1 --> 2 --> 4 --> 9 --> 18 --> 20,21 [1/256]
The chart shown for Carlos II is more than adequate to display his extreme
inbreeding, but he has other Habsburg ancestors.
#110's mother is the 1-g granddaughter of #256.
#45's father is the 1-g grandson of #256;
#45's mother is the 2-g granddaughter of #256,
and the 2-g granddaughter of #340 (agnatic nephew of #512 and
grandfather of #85).
These additional connections give Carlos II a total of 40 lines of descent from
Albert II Habsburg; this grows as we go farther back:
106 lines from Albert I and 387 lines from Rudolph, the first Habsburg Emperor.
Go back to the pedigree start page.
Go back to my home page.